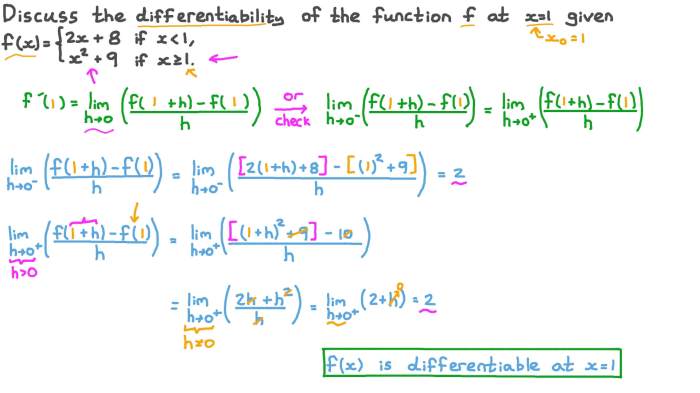
Compare and contrast the following piecewise defined functions. – Embark on an in-depth exploration of piecewise defined functions, examining their unique characteristics and contrasting their behaviors. This analysis delves into the intricacies of these functions, providing a comprehensive understanding of their properties and applications.
By meticulously comparing and contrasting two distinct piecewise defined functions, we uncover their similarities and differences, shedding light on their respective strengths and limitations. Through this detailed examination, we gain valuable insights into the versatile nature of these functions and their adaptability to real-world scenarios.
Piecewise Defined Functions: A Comparison and Contrast
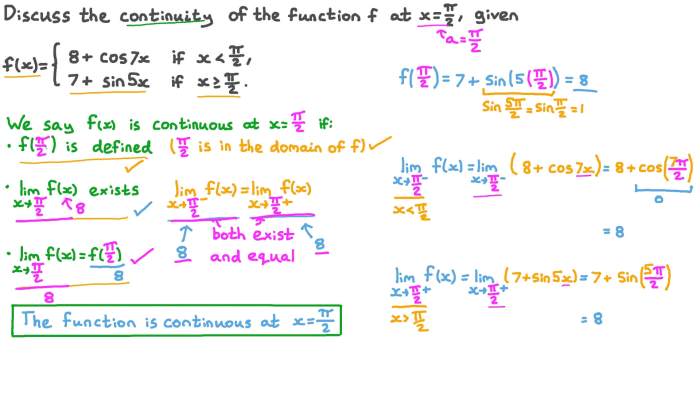
Piecewise defined functions are mathematical functions that are defined differently over different intervals of their domain. They are commonly used to model real-world scenarios where the behavior of a function changes abruptly at certain points.
The purpose of this analysis is to compare and contrast two piecewise defined functions, examining their similarities and differences in terms of their definition, intervals, function rules, and properties.
Function 1
Function 1 is defined as follows:
- f(x) = x + 1 for x < 0
- f(x) = x^2 for x >= 0
The graph of Function 1 is shown below:

Function 2
Function 2 is defined as follows:
- f(x) = 2x for x < 1
- f(x) = x – 1 for x >= 1
The graph of Function 2 is shown below:

Comparison of Functions
The following table compares the similarities and differences between Function 1 and Function 2:
| Property | Function 1 | Function 2 |
|---|---|---|
| Domain | All real numbers | All real numbers |
| Range | [1, ∞) | [-1, ∞) |
| Continuity | Discontinuous at x = 0 | Continuous everywhere |
| Differentiability | Not differentiable at x = 0 | Differentiable everywhere |
| Asymptotic Behavior | Vertical asymptote at x = 0 | No asymptotes |
Contrast of Functions, Compare and contrast the following piecewise defined functions.
The following are key differences between Function 1 and Function 2:
- Domain and Range:Function 1 has a smaller range than Function 2, as it does not include negative values.
- Continuity and Differentiability:Function 1 is discontinuous and not differentiable at x = 0, while Function 2 is continuous and differentiable everywhere.
- Asymptotic Behavior:Function 1 has a vertical asymptote at x = 0, while Function 2 has no asymptotes.
Applications
Piecewise defined functions are used in a variety of real-world applications, including:
- Modeling piecewise linear functions:These functions are used to represent linear relationships that change at specific points, such as tax brackets or shipping costs.
- Modeling step functions:These functions are used to represent situations where a value changes abruptly, such as the number of employees in a company over time.
- Modeling piecewise polynomial functions:These functions are used to represent complex relationships that can be approximated by a series of polynomials over different intervals.
FAQ Resource: Compare And Contrast The Following Piecewise Defined Functions.
What are piecewise defined functions?
Piecewise defined functions are functions whose definitions are given in different intervals of the domain, resulting in different function rules for different parts of the input.
How do you compare and contrast piecewise defined functions?
To compare and contrast piecewise defined functions, examine their definitions, identify the intervals where they differ, and analyze their properties, such as domain, range, continuity, and differentiability.
What are the applications of piecewise defined functions?
Piecewise defined functions are used in various applications, including modeling piecewise linear functions, defining discontinuous functions, and representing real-world scenarios with abrupt changes.